Ellipses
1. Mathmatical Definiton : "Set of all points such that the sum of the distance from two points is a constant."
- 2. Algebraically Definition: An ellipse can either be "fat" or "skinny". The equation for a fat graph is (x-h)^2/a^2 + (y-k)^2/b^2 =1, the bigger number "a" being on the bottom of x. If the graph is skinny then the equation of the graph is (x-h)^2/b^2 + (y-k)^2/a^2 =1, the bigger number "a" being below the y in this instance.So what you have to do is plot given information and find out which points are vertices and which ones are co-vertices.
- From this, you should be able to identify a and b. Remember that the vertices lie on a long the major axis, which is the longer one. If given the focus and either the vertices or co-vertices, use equation c^2=a^2-b^2 to find missing value. From given information, find center (h,k). Put a^2 and b^2 into general equation. Make sure they are underneath the correct term (if major axis vertical, a goes under the y^2 term; if major axis is horizontal, a goes under the x^2 term). (Kirch)
The eccentricity is a measure of how much the conic section deviates from being circular. The eccentricity for an ellipse is 0<e<1. "An ellipse is defined in part by the location of the foci. However if you have an ellipse with known major and minor axis lengths, you can find the location of the foci using the formula below. The major and minor axis lengths are the width and height of the ellipse." (http://www.mathopenref.com/ellipsefoci.html)
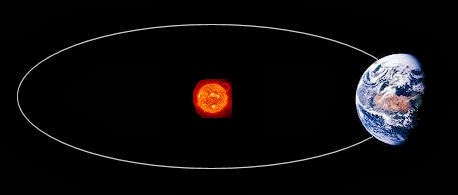
3. Real World Application
As we create the ellipse we have to take in consideration that we can't skip the equation of the ellipses c^2=a^2-b^2 to find the missing value. Remember that "c" is the focus of the ellipses.
The Video Is Here: www.youtube.com/watch?v=6pDh42E2bbA :)
Here in this picture we see that that Earth actually revolves around the sun in an ellipse motion and it isn't perfectly circular. "The radius of this orbit is 150 million km (which is, of course, the distance to the Sun.) and it takes a YEAR (365¼ days), for the Earth to complete ONE orbit, to fit this into the calendar we have 365 days for three years and then 366 days for a leap year." (www.telescope.org/nuffield/pas/earth/earth5.html)
http://en.wikipedia.org/wiki/Ellipse
4. Citations
(www.telescope.org/nuffield/pas/earth/earth5.html)
www.youtube.com/watch?v=6pDh42E2bbA
http://www.mathopenref.com/ellipsefoci.html
(www.telescope.org/nuffield/pas/earth/earth5.html)
www.youtube.com/watch?v=6pDh42E2bbA
http://www.mathopenref.com/ellipsefoci.html
http://en.wikipedia.org/wiki/Ellipse

No comments:
Post a Comment